How to teach Special Relativity

I am convinced that the way relativity is taught is all wrong.

Describing the SAME EVENT through different viewpoints

It is the SAME event that is being described by both the observers. This event can be the hand of a ticking clock, the explosion of a firecracker or anything else.

For starters, we always assume our objects, be they spaceships or moving trains, to be in ‘flat space’. This very starting point in our ‘visualization’ is incorrect. Space is not flat. Not near the Earth’s Surface or anywhere else in the Universe!

Two thought experiments were key to my understanding, and being able to visualize, how the ‘magic’ of the Special Theory actually worked.

Traveling alongside a beam of light (thought experiment via Einstein)

If you were to move alongside a beam of light, and you were slowly speeding up, you would expect that the light beam would move slower relative to you. As an extreme case, when you were moving at the SAME speed as the light beam, you should see it as stationary.  Of course, this is exactly the thought experiment that tortured Einstein.

For light to even ‘appear’ stationary,  maxwell’s electromagnetic equations (equations that applied to light waves), would need to have a ‘standing wave’ solution. I.E. – instead of always providing traveling waves as the answer, for some cases, the equations needed to provide stationary waves (which are different mathematical objects) some of the time.

Maxwell’s equations provided no such escape.

Hence, as bizarre as it sounds, when you travel alongside the beam, AT nearly the same speed – light STILL moves at the speed of light!

How can that even be physically possible? Einstein realized that the only way this could even be possible was if YOUR ruler and YOUR clock also differed from the ruler and clock of the light beam.

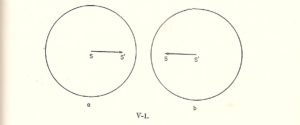
Can a sphere have 2 different centers?

From wikipedia, the section below on Menzel’s spheres

An elegant solution to the paradoxical difficulties is apparently achieved by Donald H. Menzel in his book Mathematical Physics, pages 376 and 377

Menzel describes the problem basically in the same way as does Muirhead, but he adds, “It seems paradoxical even to suggest that the form of the wave front as viewed from S’ could be considered by that observer as a sphere centered at S’.

But that is exactly what the relativity postulate requires. The velocity of light, as measured in either system must be a constant. There is no essential difference between S and S’ since both are inertial systems.

Thus figure 1b presents the reciprocal conditions from the viewpoint of observer S’.”

Figure 1b is drawn as two separated circles, with separated origins S and S’, which are moving in opposite directions. Menzel continues: “Certainly we cannot possibly satisfy (5) and (6), which imply the existence of one sphere with two different centers, if we retain Euclidean ideas of measurement. We have already seen, however, that the the classical concept of simultaneity and therefore of a universal time system runs into difficulties. Perhaps spatial conditions are similarly altered…”

End wikipedia extract.

Understanding Relative Time – Muons and Time Dilation

  A muon that lives for a fraction of a second in a lab, survives for minutes on it’s way through the Earth’s atmosphere, as it travels close to the speed of light. What gives? How can something that is only supposed to live for a second, suddenly live for a 1000 times longer than that?

I often struggled with visualizing things like ‘length contraction’ and ‘time dilation’.

What does simultaneous mean? There’s no such thing as ‘Simultaneous for everyone’. If a train passes a station at 10:00 AM, that is actually TWO events. The train passing and a clock striking 10. These two events may seem simultaneous to an observer below the clock, but they will not be simultaneous for a passenger on the train. In fact, the passenger sees the clock at 10:0X – a little later than 10:00  AM – since it takes a little longer for the light from the clock to reach the moving passenger!

Understanding Relative Lengths – Bell’s spaceship experiment and the rod in the barn

Speeding Rod through an open barn

For the farmer in the barn, the rod DOES fit into the barn as he shuts both the front and the back door (simultaneously, as he sees it).

For the spaceman on the rod, the rod also sees both the doors close – but at different times. First, the back door of the barn closes – and then the front door – trapping the bar within. There is no discrepancy here, if we remember this almost central consequence of relativity – simultaneous events in one frame aren’t simultaneous in another.

The same physical events MUST occur (both the doors must shut) –  but the events do not have to have the same time ordering for all observers.

Proper Length

Reference Frames

Next Steps?

The Physics Class you wish you had